- Momentum:- Momentum of a particle (p) is equal to the mass of the particle (m) times velocity of the particle (v). So, p = mv
- Newton laws of Motion:-
First Law (Law of Inertia): Everybody continues in its state of rest or of uniform motion in a straight line unless it is compelled by some external force to change that state.
Second Law: The rate of change of momentum of a body is directly proportional to the impressed force and takes place in the direction of the force.
So, F= dp/dt
This results force acting a body F is equal to the mass of the body m times acceleration of the body a.
So, F = ma
Third Law: To Every action there is an equal and opposite reaction.
FAB = - FBA
- Impulse:- Impulse of a force is defined as the change in momentum produced by the force and it is equal to the product of force and the time for which it acts.
So, J = Ft
= mv – mu
- Apparent weight of a man inside a lift:-
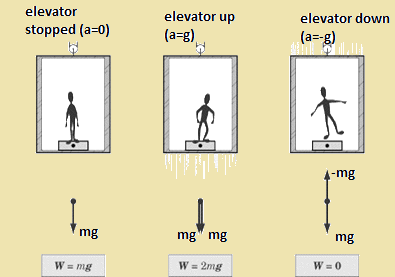
(a) The lift possesses zero acceleration (fig-1): W = mg
(b) The lift moving upward with an acceleration a (fig-2):
W = mg + ma
= mg + mg
= 2 mg
(c) The lift moving downward with an acceleration a (fig-3):
W = mg – ma
= mg – mg
= 0
- Inertial mass (mi)- It is defined as the ratio between force F to the acceleration a a produced in the body.
So, mi = F/a
Note: When the velocity of a body is comparable to the velocity of light, inertial mass changes with velocity in accordance with following formula,
mi = mi0/√(1-v2/c2)
Here mi0 is the rest mass of body, v is the velocity of body and c is the velocity of light.
- Conservation of linear momentum:- In an isolated system (no external force), the algebraic sum of the momentum of bodies, along any straight line, remains constant and is not changed due to their mutual action and reaction on each other.
- Recoil of gun:- If m is the mass of the bullet, M is the mass of gun and v is the mass of the bullet, then, velocity of the recoil of gun V will be,
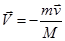
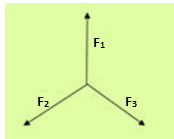
- Equilibrium of concurrent forces:-
Forces are said to be concurrent if they meet at a common point. Below figure shows three concurrent forces  ,
,  and
and 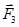 .
.
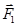 ,
, 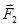 and
and 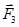 .
.
- Moment of the force = F×d
Here F is the force and d is the distance of line of action.
- Momentum of a couple (Torque (
 )):- Momentum of a couple is equal to the product of force F and the perpendicular distance r between them.
)):- Momentum of a couple is equal to the product of force F and the perpendicular distance r between them.
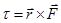
- Work done by a couple: (W)-
W =  × θ
× θ
 × θ
× θ
Here 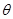 is the angular displacement.
is the angular displacement.
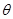 is the angular displacement.
is the angular displacement.- Connected motion (Pulley problem):-
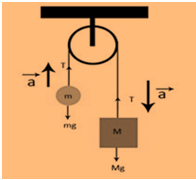
(a) Driven body moving vertically:-
Acceleration of the system, a = (M-m/M+m) g
Tension in the string, T = (2Mm/M+m) g
The force on the pulley, F = (4Mm/M+m) g
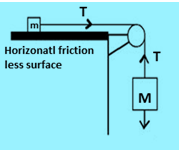
(b) Driven body moving horizontally:-
Acceleration of the system, a = (M /M+m) g
Tension in the string, T= (Mm/M+m) g
The force on the pulley, F= [√2 (Mm) /M+m] g
Here g is the free fall acceleration.
- Application of Newton’s third law on contact of two block:-
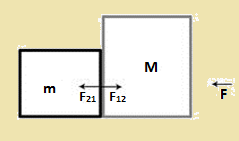
(a) Acceleration, a = F/(m + M)
(b) 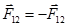
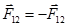
(c) Contact force, F12 = (m/m+M) F = F21
- ?Horse and Cart Problem:-
A horse pulls a cart with a certain force while the cart exerts the same force on the horse in opposite direction.
If P is the horizontal component, F is the force of friction which opposes the motion of the system, m1 is the mass of the horse and m2 is the mass of the cart, then the acceleration of the system will be,
a = P – F/m1+ m2
This equation indicates that the motion of the system will take place so long as P is greater than F. Existence of equal and opposite tensions in the string creates no hindrance to the motion.
- Friction:- Whenever a body tends to slide over another’s surface, an opposing force, called force of friction comes into play. This force acts tangentially to the interface of two bodies.
- Static friction:- Static friction is the force of friction between two surfaces so long as there is no relative motion between them. It is always equal to the applied force. The static frictional forces are incorporated in the following inequality.
fs ≤ µsN
- The magnitude of static friction fs (static frictional force) has a maximum value fs,max that is given by,
fs,max = µsN
Here µs is the coefficient of kinetic friction and N is the normal force.
So, coefficient of static friction, µs= fs,max /N
- Kinetic friction:-
? Kinetic friction is the force of friction which comes into play between two surfaces when there is some relative motion between them. The magnitude of force of kinetic friction fk (kinetic frictional force) is proportional to the normal force N.
So, fk = µk N
Here µk is the coefficient of kinetic friction.
Thus, coefficient of kinetic friction, µk = fk/N
- µk ?µs
- Rolling friction:-
Force of friction which comes into play, between two surfaces, while one is rolling over the other is called rolling friction. Rolling friction is similar to kinetic friction.
So, fr = µr N
Here µr is the coefficient of rolling friction and N is the normal force.
- Angle of Friction:-
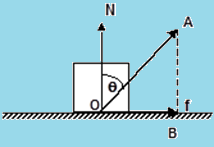
The angle made by the resultant reaction force with the vertical (normal reaction) is known as the angle of the friction.
Now, in the triangle OAB,
AB/OB = cotθ
So, OB = AB/ cotθ
= AB tanθ
Or, tanθ = OB/AB
= f / N
So, tanθ = f / N = µs
- Angle of Repose:- It is the angle which an inclined plane makes with the horizontal so that a body placed over it just begins to slide of its own accord.

Consider a body of mass m resting on an inclined plane of inclination θ. The forces acting on the body are shown – Ff being the force of friction. If friction is large enough, the body will not slide down.
along x: mg sin θ – f = 0 …(1)
Along y: N –mg cosθ = 0 …(2)
i.e. N = mg cos θ and f = mg sin θ
Thus,  gives,
gives,
 gives,
gives,
mg sin θ ≤ 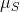 mg cos θ
mg cos θ
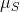 mg cos θ
mg cos θ
So, tan θ ≤ 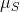 . This signifies, the coefficient of static friction between the two surfaces, in order that the body doesn’t slide down.
. This signifies, the coefficient of static friction between the two surfaces, in order that the body doesn’t slide down.
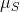 . This signifies, the coefficient of static friction between the two surfaces, in order that the body doesn’t slide down.
. This signifies, the coefficient of static friction between the two surfaces, in order that the body doesn’t slide down.
When q is increased, then tan θ > 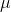 . Thus sliding begins, and the angle θr = tan-1
. Thus sliding begins, and the angle θr = tan-1 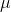 . This angle is known as the angle of repose.
. This angle is known as the angle of repose.
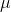 . Thus sliding begins, and the angle θr = tan-1
. Thus sliding begins, and the angle θr = tan-1 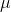 . This angle is known as the angle of repose.
. This angle is known as the angle of repose.- Acceleration on a horizontal plane:- a = (F - µkN)/m
- If
 be the acceleration of frame, the pseudo force will be,
be the acceleration of frame, the pseudo force will be,
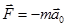 (Example: centrifugal force = mv2/r = mrω2)
(Example: centrifugal force = mv2/r = mrω2)- Gravitational mass (mg):-
mg = F /g
= FG r2/GM
Here, g is the free fall acceleration, FG is the gravitational force and G is the gravitational constant.
written by arpan ruhil
good notes
ReplyDelete